Statıstıcs I Deneme Sınavı Sorusu #841397
Let E1, E2, ..., Ek be a collection of mutually exclusive and collectively exhaustive events. Then for any event A with P(A)=?0 and any i=1, 2..., k
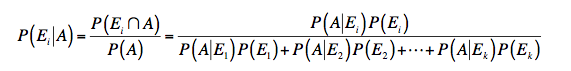
What is the formula above?
Let E1, E2, ..., Ek be a collection of mutually exclusive and collectively exhaustive events. Then for any event A with P(A)=?0 and any i=1, 2..., k
What is the formula above?
|
Multiplication rule |
|
Bayes’ Theorem |
|
Random experiment |
|
Elementary outcome |
|
Independence probability |
Yanıt Açıklaması:
An important application of conditional probability is given in the following result, which is known as Bayes’ Theorem.
Let E1, E2, ..., Ek be a collection of mutually exclusive and collectively exhaustive events. Then for any event A with P(A)=?0 and any i=1, 2..., k
P(Ei A)=P(Ei?A)= P(AEi)P(Ei)
P (A) P (A E1 )P (E1 )+ P (A E2 )P (E2 )+!+ P (A Ek )P (Ek )
Yorumlar
- 0 Yorum


